Digital surface regularization by normal vector field alignment
Abstract
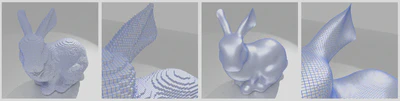
Digital objects and digital surfaces are isothetic structures per se. Such surfaces are thus not adapted to direct visualization with isothetic quads, or to many geometry processing methods. We propose a new regularization technique to construct a piecewise smooth quad- rangulated surface from a digital surface. More formally we propose a variational formulation which efficiently regularizes digital surface ver- tices while complying with a prescribed, eventually anisotropic, input normal vector field estimated on the digital structure. Beside visualiza- tion purposes, such regularized surface can then be used in any geometry processing tasks which operates on triangular or quadrangular meshes (e.g. compression, texturing, anisotropic smoothing, feature extraction).
@inproceedings{coeurjolly17regDGCI,
address = {Vienna, Austria},
author = {Coeurjolly, David and Gueth, Pierre and Lachaud, Jacques-Olivier},
booktitle = {20th International Conference on Discrete Geometry
for Computer Imagery},
doi = {10.1007/978-3-319-66272-5_17},
hal_id = {hal-01543007},
hal_version = {v1},
keywords = {digital geometry ; surface regularization ; normal
vector field},
number = {10502},
pages = {197--209},
pdf = {https://hal.archives-ouvertes.fr/hal-01543007/file/article.pdf},
series = {20th International Conference on Discrete Geometry
for Computer Imagery},
title = {Digital surface regularization by normal vector field alignment},
url = {https://hal.archives-ouvertes.fr/hal-01543007},
volume = {LNCS},
year = {2017}
}